filtrability test
Reading time:büchner vacuum filterability test
measurement of the specific resistance of a sludge in a 0.5 bar vacuum.
This test is typically used to estimate a filter filtration capacity for industrial vacuum applications. It can also be used to establish the optimum amount of reagents that are required for belt filtration, however in this case allowance should be made for the compressibility factor.
Photo 7 displays the test apparatus required (removable büchner, filtering medium consisting of a rapid filter paper).



operating method
Fill the büchner with sludge (pretreated), 100 to 150 mL of sludge is usually sufficient to produce an 8-10 mm thick filter cake.
Create the vacuum to quickly reach the level required, i.e. 1.5 bar, ensuring that this level remains constant throughout the test.
As soon as the vacuum has been created, start the stop- watch and record the volume of filtrate collected. Substract this initial volume V0 at time t = 0 from later volumes recorded. Log the volumes of filtrate collected during the test for various filtration times: every 10, 15, 20, 30 or 60 seconds depending on the filtrate flow rate. Pursue the filtration until the cake is drained (loss of vacuum caused by the cake cracking up).
calculating specific filtration resistance
Filtrate volumes V0 – V1 – V2 – V3…, for times T0 – T1 – T2 – T3…, are recorded.
Plot

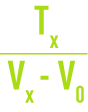
versus Vx. In principle, these points should form a straight line (except at the start of filtration and during the draining stage). The slope for the linear portion of the curve obtained provides coefficient a (figure 32, numerical characterisation of filterability). The following equation provides the specific filtration resistance at 0.5 bar (49 × 103 Pa):

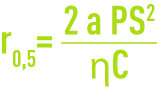
a: expressed in s · m–6,
P: in Pascal (i.e. 49 × 103 Pa),
S: in m2,
η: in Pa · s,
(at 20 °C, in the region of 1.1 × 10–3 Pa · s),
C: in kg · m–3,
r: in m · kg–1.
Note: C, dry solids content at 105°C divided by the volume of sludge, is an approximation of W (mass of suspended solids deposited per unit of volume of filtrate).
pressurised filtration suitability test
A pressure cell (figure 20) is used to determine the specific filtration resistance, the compressibility coefficient and the limit dry weight of filtration cakes. The principle described in § 6.6.1. also applies to this method.

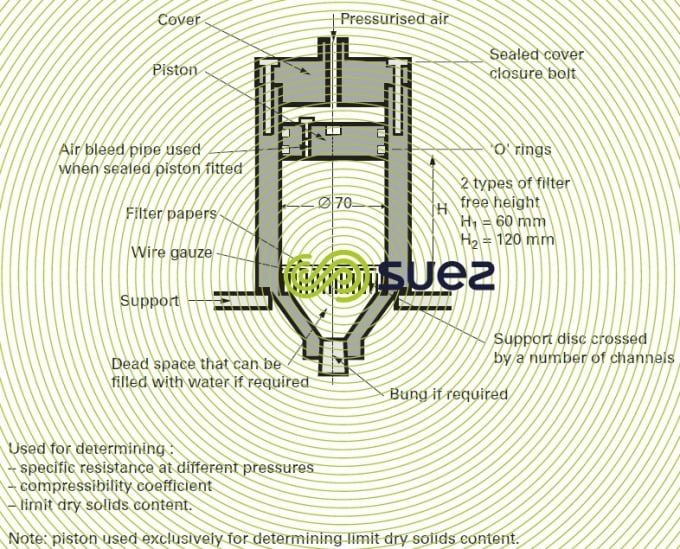

operating method
- dampen the paper filter and apply slight excess pressure in order to check the cell floor for sealing and to remove any excess water held in the filter;
- position the test tube beneath the cell’s funnel;
- pour the sludge sample (100 to 150 mL) into the cell;
- allow to rest for 15 seconds before applying pressure to form a precoat on the filter.
- gradually apply the pressure selected (0.1 to 15 bar); the use of the piston is not recommended for pressures below 2 bar;
- allow the filtrate to flow and record its volume V0 (approximately 10 % of the volume of the sludge to be filtered);
- start the stopwatch and record filtrate volume V according to time.


The rate at which readings are collected depends on the filtrate flow rate.
Please refer to (filtrability test) for information on calculating the resistance.
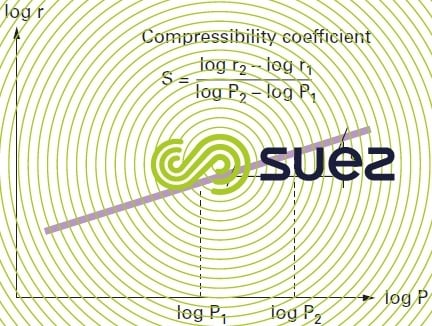
determination of the compressibility coefficient (figure 21)
Measure the filtration specific resistance r for different pressures P and plot the curve log r = f (log P). Check the linearity. The slope is equal to the compressibility coefficient.


The same apparatus as for the preceding test is used.
It is recommended to use regular increment in pressure for maximum precision. The following figures are recommended:
P = 49 kPa – 147 kPa – 441 kPa
and 1 323 kPa (or maximum pressure tolerated by laboratory equipment).
The compressibility coefficient is a non-dimensional number.
Bookmark tool
Click on the bookmark tool, highlight the last read paragraph to continue your reading later












